Stories

Stories below illustrate teachers getting excited by the tools or methods used in Calculating Changes just as Nicholas Dale does in his article Threading Works which features in the In Brief link. Members have access to more stories. If you would like to contribute to these stories, email Doug.Williams: doug@blackdouglas.com.au

You will also find stories about involving children in mathematical investigations which model working like a mathematician in the Research & Stories section of Mathematics Centre.

Karren Jennings
Caledonian Primary School, Ballarat, VIC

This story is about fascinating, captivating and absorbing kids in mathematics. It was contributed just 3 months after the school joined the Calculating Changes network. Karren organised the professional development day and parent evening which introduced staff to Calculating Changes and other aspects of learning to work like a mathematician. You can read about the PD day too.

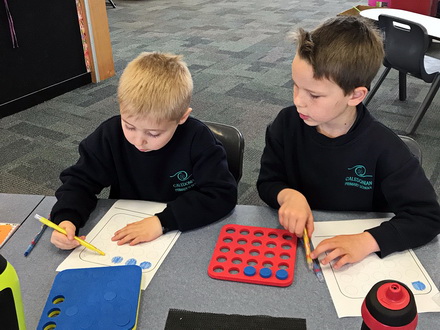I was coaching in the Preps' classrooms and both classes have been doing the Plug Snakes activity. The photos are a mix from both classes - Kate McEldrew (Miss Kate) and Mandy Polkinghorne (Mrs P.).

I have attached the recording the students have been working on this week. It has been fantastic to see them up-level their recording, renaming and grouping plugs!

The students recorded their 'snakes' on the frame photocopy and wrote a number sentence to match.

Then they found ways to group the plugs to write a 'simpler' sentence. Many worked out they could make groups of ten!

One photo shows the model on the whiteboard. I know the teachers are quite excited and intend to go on with this next week.

Editor's Notes

1. The faces show the children are certainly fascinated, captivated and absorbed. Karren's words and the teachers' intention to continue confirm it.

   Teacher Challenge:
   Re-read the photos and words with a colleague. Try to identify 7 reasons why these children are so involved and appear to be doing maths that some might not expect at this age and stage.

2. In one photo there is a calculator on the table. How might it be used? What could using a calculator add to the possible learning in the activity?

3. Calculating Changes members can learn more about Plug Snakes.

Lisa Harris
St. Vincent's Primary School, Aranda, ACT

I wanted to share something that worked really well for me in my classroom last week.

Two of the big focuses for our school this year are Numeracy and differentiation. After a bit of reading about differentiating in the mathematics classroom, as well as combining what I learnt from your sessions (see below); I wanted to come up with a lesson that all students would be successful with.

One article I read said, ...effective differentiation can be as simple as turning a text question into an open ended question! ... so I did this:

Instead of presenting a word problem such as I have 2 paddocks with 4 cows in each paddock, how many cows all together?, I said I want you to create a problem involving animals and paddocks. At first I got a few blank faces but then once we got started the responses from the students blew me away.

• Some children drew paddocks with animals in them and asked how many animals all together?
• One student wrote a narrative about how she had inherited land and money and had to build a farm using what she had been given.
• Another student measured the perimeter of all her paddocks and then I posed the question What happens if I have to remove some of the fencing and put in a gate?.

I believe the responses could be limitless and it wouldn't matter if the responses are simple or complex it still requires a high degree of higher order thinking. Also, there are so many different mathematical concepts that could be covered in that one open-ended task.

The fire in my mathematics belly has been reignited after returning from the summer break!!

Editor's Notes

1. Through the previous year, Lisa and another teacher from Aranda enjoyed a six day professional development program from Mathematics Centre that was arranged through the Catholic Education Office Canberra Goulburn. This was the third year of the program, during which time representative teachers from every school in the Diocese were involved in the six days.
2. Derek Holton & Charles Lovitt have published Lighting Mathematical Fires 2 through Curriculum Press. It is for teachers of Years 2 - 8 and could help you to ignite your belly fire too.
3. Many of the Calculating Changes activities have this open feel about them that encourages children to do more than you expect. If you are just getting to know the network, we encourage you to explore the Free Tour Activities and look for the aspects of each that give learners a choice and encourage ownership of the mathematics.

Shane Hoffman
Clunes Primary School

Clunes Primary School began using Working Mathematically with Infants towards the end of Term 1. This program interweaves threaded activities and investigations. Crossing The Desert is an investigation suggested for Year 2. It is explained in the manual but there is information about it in the Task Cameo for this investigation. The investigation is revisited in the Year 5/6, Space & Logic, Maths With Attitude kit.

Shane's story highlights the delights of working on investigations with young children and indicates the type of adaptation teachers make to support children in this type of learning. Given the physical involvement adaptation in Cat & Mouse below, teachers might consider exploring the problem in larger scale on the floor as a class introduction before letting groups loose with their Poly Plug.

The task however, perhaps due to its open-endedness, was extremely challenging for this particular age group. Working in pairs and using Poly Plug, the students were very persistent and came up with many different theories early on. In fact the questions they were generating were nothing short of hilarious! I was constantly bombarded by queries such as:

• Who are they delivering the message to? Couldn't this person just give the travellers enough food to make it home?
• Why don't they just send an email or text message instead of risking their lives to deliver a message to someone who only has enough food for themselves?

While their ability to think outside the square was admirable, it was obvious that I needed to provide plenty of support throughout the lesson in the form of Handy Hints such as:

• Do both travellers necessarily need to complete the entire journey to deliver the message?
• The travellers can take one extra item with them: a shovel!

Due to time constraints and the difficulty of the task it was clear that the chance of a group solving the problem was unlikely, so I decided to conduct a 'Fishbowl' and provide them with one of the five solutions. Students were then given enough time to replicate this solution and describe their understandings.

Last week I thought it would be a good idea to revisit this activity to see how much the children remembered and whether they would be able to solve it independently. Once again we revisited the story and the problem the travellers had. Again students worked in pairs, however this time they were to imagine that they and their partner were the actual travellers. This meant that students were more likely to ensure that they both made it home safely with just enough food to spare.

This second time around the lesson was much more efficient because the students were aware from the beginning that the key to this task lies in burying excess food stocks. Once again I was overrun by theories that did not quite solve the problem safely. In general, students were able to deliver the message and get one traveller back to safety; however the other traveller was often left to perish in the desert! Because of this, the new focus for the lesson became not only burying food, but the giving of food from the burier to the messenger. Thankfully three or four pairs were able to derive a plausible solution, given this extra support, and were able to explain the theories to their peers. At the end of the lesson I once again conducted a 'Fishbowl' and revealed all five possible solutions. The students were fascinated that there were so many methods of solving the problem, yet I felt that most of them clearly understood that the concept of burying and giving were crucial. This activity may be better suited to Year 3 to 6 students, but I highly recommend giving it a try in the early years also, as long as plenty of support is provided. Fellow teachers, it is a good idea to attempt this task on your own beforehand and have the solutions on-hand throughout the lesson!

Robyn Floyd & Staff
Glen Iris Primary School

Each black pathway indicates which way to jump depending on whether an odd or an even number is rolled. The adaptation of the task to this physically-involving floorboard size is the sort of teaching craft insight that flows through all 45 Investigations described in the manual.

The 60 weeks of planned activity in the manual develops number sense, number concepts and number skills through Threaded Activity, while simultaneously putting the number sense to work through problem solving Investigations, just as a mathematician would.

Doug. Williams
Project Manager, Mathematics Centre

Counting Frames

In Meekatharra, Western Australia, I was working in a K-2 class. It was their first time with Poly Plug so I started with Free Play. That went well - each pair around the circle made something different and I was able to challenge each with a question or two based on their own exploration. My plan was to move from here to a more structured activity and I had chosen Counting Frames. I wanted to find out how these young ones would go counting by twos.

Using my own red board as an example I asked each pair to make this:

Then, thinking to myself what a wonderful teacher I was, I stuck my fingers two at a time through the gaps and we counted happily to 10 by twos. Next I called on the mathematician's question What happens if...?:

Now suppose we put all our boards on the floor side by side. Like this...

...and make a sort of road train, can we count the gaps now?

But now the kids were hooked. The collective drawing in of breath at the challenge of counting all these windows by twos was audible, but they were excited. So was I.

It lasted about four milliseconds. One Grade 1 boy went 2, 4, 6, 8, 10 ... 20, 30, ... and the others all went Oh yeah.

Once again I had been too focussed on what I wanted to teach and had risked being insufficiently aware of what the children had to offer.

Highest Number

But being the winner wasn't enough. The pair had to work out by how much the winner won. And, calling again on the Working Mathematically process, had to check their answer another way. They used materials in the room such as MAB 10, Unifix and calculators and displayed insightful mental arithmetic with comments like: It would have been 100 because of that column but the ones column is 6 for the winner and 8 for the loser, so the total is 2 less, so 98.

The children enjoyed the game much more because of the added challenges and the learning was much deeper than it would have been had I simply expected them to keep track of how many times each person won.

Robyn Anderson
Principal Project Officer, Mathematics, Metropolitan Region, Queensland

I facilitated a workshop at the QAMT Early Years conference It all adds up last Saturday 15th May 2010. The title was Bits and Bobs: A Collection of Activities to Teach Problem Solving Skills. Problems lead students to search for and find patterns in mathematics. This workshop explores ways to assist students to develop deep mathematical knowledge, reasoning ability and communication skills to investigate. I used Working Mathematically with Infants and lots of Poly Plug, dice, calculators, computers, and other 'maths stuff'. The theme of the conference explored where play meets the curriculum. Poly Plug was an excellent medium to help the teachers in the workshop to remember how to play again. We had some amazing 3D constructions with moving parts! The mathematician's question Can I check this another way? elicited a lot of thinking as the teachers had not been ensuring their students had more than one way of working when problem solving. In particular this enables comparing and justifying, important ways of working for thinking and reasoning. The learning activities in WMI model multiple materials. There ensued a lengthy discussion about access to: calculators as learning tools paper and pens for free drawing a range of materials to model dice computer drawing programs virtual manipulatives and mind-mapping programs.

All in all the 30-40 participants in each workshop were engaged and positive about the activities and Poly Plug and worked to establish how it might best fit with their planning for young students developing problem solving skills. 'Inspiring' and 'interesting' were among the comments and feedback from the participants.

July 2010

Also did a Problem Solving workshop with your materials at QAMTAC 2010 Functioning Mathematically. I drew the last workshop slot of the conference but still had 18 attend. My thesis was that we must teach students to make choices - empower them to make the choices as to how they are going to solve problems - as against the teacher knowing the 'best' or often only 'one' way and then remembering it.

I used several investigations from WM with Infants which confounded the secondary teachers when the source was revealed. The participants got to choose from:

• Ten Friends, Ten Tens (Free Tour Activities)
• Uncover Counting
• Rows & Straws - they all got excited about the extending potential of arrays
• Luke's Fraction Game - ...don't get me started on lack of understanding of the importance of early fractional understandings
  (Member Activity submitted by Sue Davis who tells her story below)
• Fill The Board (Member Activity)
• Visual = Number (Member Activity)

I have loved doing these workshops with your materials. There is so much work still to be done. If the Australian Curriculum can drive the agenda for the necessity of frequency, and access, for teachers to good quality professional learning in mathematics pedagogy, bring it on!

Sue Davis
G.A.T.E.Ways Teacher

WEEK 1
Exhausted, Yes! Exhilarated, Yes!

I have just returned from teaching a bright bunch of GATEWays children in a 2 ½ hour workshop using Poly Plugs. I began asking the children, "What do Mathematician's do?". One of their responses was that Mathematicians work out the hardest problems of all.

I wrote a problem up on the board and asked them to read it and have a go. Some of the children looked a little perplexed and stated that they had read it but did not understand what it meant. I explained that this happened to Mathematicians too, but they had a strategy to help them. This lead naturally to the first Problem Solving Strategy: Read to understand the problem. They recorded this in their books.

Taking their Poly Plugs out of the bags, we then reread and understood what the problem was and so the children progressed enthusiastically into creating the numerals 0 to 9 on the Poly Plugs and recording them on Poly Plug paper. (See the activity Making Digits, Free Tour Activity.) They were bubbling when they recognised that these were the numerals they saw on their digital clocks and calculators.

After the break, we began exploring examples of symmetry found in nature and in the classroom. I asked them to create symmetrical patterns on their Poly Plugs feeling that when they understood this we would move onto rotational symmetry. (See the activities Exploring Symmetry and Rotation Challenges, both Free Tour Activities.) Before I had the chance, the children were bringing symmetrical patterns to me and exhibited such astonishment that when they rotated their Poly Plugs, their patterns remained symmetrical. Who was leading who in this investigation!!

We then moved onto a challenge using the task Row Points. I asked the children to turn over 13 plugs on the yellow/blue board. Writing the problem on the board, the children were encouraged to use the first Problem Solving Strategy. We clarified the scoring of the task and a few rules. I also wrote the challenges on the board that included:

• What is the highest possible score?
• What is the lowest possible score?
• Are there any scores between the lowest and highest scores that can't be made?
• What scores do symmetric designs get?

One lad scored his design and despondently advised that he scored zero. It was great to see his transformation when I congratulated him. I asked him which of the challenges he had solved. The children buzzed between their tables and the board as they created new designs and improved their scores. We ended the workshop on such a high!

I have come home to rewrite next week's session as the excitement and enthusiasm just has to be allowed to continue with the obvious opportunities to go deeper into the investigation. Just how many designs are there which can score zero??

Calculating Changes ... is a division of ... Mathematics Centre