
Doug's TableclothTask 3 ... Years 4 - 8SummaryThe problem appears simple. Fold the given piece of cloth to fit exactly into the depth of the given 'drawer' pictured on the card. However, partly because of most people's pre-disposition to fold things in half, the problem is more challenging than it first appears. Measurements and other information are provided to encourage thinking the problem through at a deeper level.This cameo has a From The Classroom section which shows children from one school exploring the task. The cameo also includes an Investigation Guide. |
Materials
Content
|

A Special History
|
This problem began with a real cloth that would not fit back into the real drawer it had come from following its first outing at a dinner party. Sally Farr, who had come from Queensland to investigate the use of tasks with Indigenous students was present as Doug tried - unsuccessfully - to refold the cloth to fit. She suggested there could be a task in this problem ... and she was right. Later, towards the end of the 1990s, Per Berggren, a well-known and respected teacher in Sweden, purchased Doug's Tablecloth to add to his school's collection of tasks (mattegömmor). He was so puzzled by it that he eventually flew to Australia to try it with the actual drawer and actual cloth which had first generated the problem at Doug's house. As you can see, it was no less puzzling 'in the flesh', but perseverance won out in the end. See below for students struggling (and succeeding!) with this task. |
IcebergA task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. |
The strategy of working backwards plays a big part in this task:
One way to tell someone else how to do it (and thereby stopping them from thinking) is:
|
Note: This investigation has been included in Maths At Home. In this form it has fresh context and purpose and, in some cases, additional resources. Maths At Home activity plans encourage independent investigation through guided 'homework', or, for the teacher, can be an outline of a class investigation.
- Visit the Home Page for more Background.
- For this specific activity click the Learners link and on that page use Ctrl F (Cmd F on Mac) to search the task name.
Whole Class InvestigationTasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. |
To convert this task to a whole class investigation each pair (or student) will need a cloth the correct size. Perhaps a parent, or the craft faculty, could cut sufficient pieces. This only has to be done once if the pieces are stored after each use. Some schools use filing boxes made from recycled cardboard to store class sets of task materials. This is an efficient use of preparation time - one preparation, multiple uses. In the future, when teachers reach the part of the curriculum document that requires a whole class investigation of a task, they simply select the appropriate box from the shelf and everything is there. Some teachers have thought of using paper instead of cloth for Doug's Tablecloth, but there is a lot of experimental folding done before the problem is solved, and paper holds its creases. Consequently, paper becomes confusing. In the long run material also retains creases, but it can easily be ironed flat again after a few uses. At this stage, Doug's Tablecloth does not have a matching lesson on Maths300. |
Is it in Maths With Attitude?Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. |
The Doug's Tablecloth task is an integral part of:
|
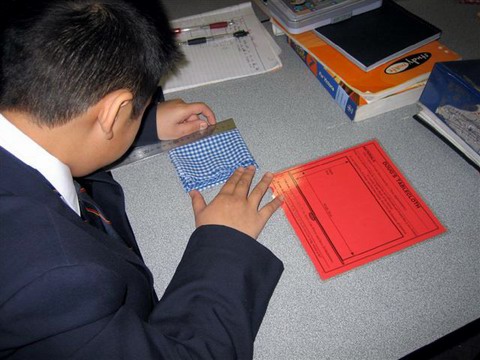
Simonds Catholic College
Helen's Class |

|
 |
 |
 |
 |