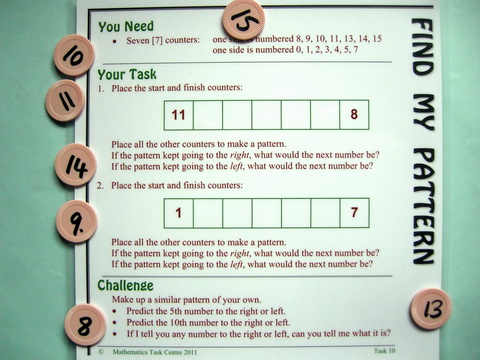
Find My Pattern

Task 10 ... Years 2 - 8

Summary

The first thing to realise about this task is that it was invented by a group of Year 4 students - so it is probably sneakier than anything a teacher could come up with! It seems simple enough. We are given:
  • The first and last numbers of a sequence.
  • The number of missing numbers in the sequence.
  • A short list of numbers from which the missing ones can be found.
All we have to do is recreate the pattern! The reasoning involved is similar to that required by archaeologists reconstructing ancient documents from a few torn samples. 		 

Materials

  • Discs with particular numbers as shown and a board with seven cells.

Content

  • basic number skills
  • recognising making and continuing patterns
  • group counting
  • generalisation
  • visual representation of patterns
Find My Pattern

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. 		   

  1. The tricky thing the Year 4 students introduce us to is a pattern with two parts. For example the solution to the first problem on the card is:
    11, 15, 10, 14, 9, 13, 8
    ... a +4/-5 pattern
  2. How many ways could this have been worked out? Perhaps guess and check. Perhaps try every possible case. But how about reasoning along these lines:
    • Somehow we have to get from 11 to 8 in a pattern using 6 steps.
    • That's an overall effect of subtracting 3.
    • If all the steps were the same we would have to subtract ½ each step.
    • Can't do that because those numbers aren't in the available list.
    • Perhaps each pair of steps is the same amount. That would still be a pattern, then each pair of steps would be equivalent to subtracting 1?
    • There could be many +/- (up/down) pairs which are equivalent to subtracting one, but of the available numbers, which is possible?
    At this point our choices are much more limited because clearly things like +10/-11 aren't going to work with the available numbers. A few trials leads to the solution above.
    Try the same reasoning for Question 2 on the card. It gets a bit more diabolical.
  3. The task includes the beginnings of working with addition and subtraction of integers.
  4. Once a pattern has been discovered, it begins to become clear that it is really two patterns, each one linking every second term. If we choose a number in the sequence to be in Position 1 and number the other positions consecutively, we can make ordered pairs (position, number). What happens if we graph these pairs?
  5. Can you predict whether zero will be in a particular pattern?
  6. What happens if we explore three step patterns?
Note: There is a strong link between Find My Pattern and Task 52, Which Floor.

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

The whole class lesson for this task is detailed in Maths300 Lesson 114, Find My Pattern. To convert the task to a whole class lesson students can quickly fold and tear or cut paper tiles like the counters. These then have to be arranged in sequence to form the pattern.

For more ideas and discussion about this investigation, open a new browser tab (or page) and visit Maths300 Lesson 114, Find My Pattern.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

The Find My Pattern task is an integral part of:

  • MWA Pattern & Algebra Years 3 & 4
  • MWA Number & Computation Years 7 & 8

The Find My Pattern lesson is an integral part of:

  • MWA Pattern & Algebra Years 3 & 4
  • MWA Pattern & Algebra Years 9 & 10

Green Line
Follow this link to Task Centre Home page.