Plate Triangles

Task 28 ... Years 4 - 8

Summary

This task needs floor space to lay out an 8-plate equilateral triangle. In the beginning plates are used to represent objects arranged in a triangle. Counting in each row generates the Natural Numbers and counting the total of plates from the beginning to the current row generates the Triangle Numbers. Now the explorations begin. Place the counters on the plates in order and look for patterns, or try the Triangle Teasers, or use the number patterns in other problems.

This cameo includes an Investigation Guide.

 

Materials

  • 36 paper/plastic plates
  • 36 counters numbered from 1 - 36

Content

  • spatial and numeric patterns
  • four operations
  • connections with algebra including generalisation of patterns
  • problem solving strategies
Plate Triangles

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. 		   

The task highlights visually and kinaesthetically that the Triangle Numbers are formed by adding consecutive Natural Numbers from 1, ie:
Sum of Natural Numbers
1
1 + 2
1 + 2 + 3
1 + 2 + 3 + 4
1 + 2 + 3 + 4 + 5
1 + 2 + 3 + 4 + 5 + 6
1 + 2 + 3 + 4 + 5 + 6 + 7
1 + 2 + 3 + 4 + 5 + 6 + 7 + 8

Triangle Number
1
3
6
10
15
21
28
36

  1. These patterns show up in many problems. For example, you might put them in context using The Twelve Days of Christmas, which is a report by a pair of Indigenous Students from Moonah Primary School, Tasmania.
  2. What is the total of the plates if we extend the triangle to 10, ...100, ...1000 rows?
  3. Can we program a spreadsheet to answer these questions?
  4. A spreadsheet can only work up to the largest number the computer can deal with. If we are going to find the total of plates for any given number of rows, we need some other way which is independent of specific numbers - a generalisation. Shapes are independent of numbers and these patterns form shapes. Explore the number patterns as shapes to try to develop a rule for calculating the total of plates no matter how many rows.
    Hint: Thousands of years ago the Greeks realised that it was easy to calculate the total of objects arranged in rectangles or squares.
  5. After placing numbers from one to thirty-six on the 8-row triangle look for other patterns. For example, sum each row. Is there a pattern in the totals, which are 1, 5, 15, 34, ...?
The task also offers some Triangle Teasers which are a little like Magic Squares. Question 9 doesn't specify which numbers to use, and there are many answers, but a mathematician likes to keep things simple if possible, so why not try using the first 6 numbers to fill the circles. Students soon realise:
  • The corner numbers are used twice.
  • The larger numbers are better in the corners.
A variety of problem solving strategies will lead to the solution:
4
3  2
5  1  6
Is there a reason why the sum of each side of the triangle is 12?

Solving Question 10, which has more clues, provides another piece of data about Triangle Teasers ... and raises more questions:

  • Why is the sum of the sides 33?
  • Could this be predicted from the numbers 8 - 13?
  • Is the solution to Q.10 related to the solution to Q.9? Arithmetically? Geometrically?
  • Can we make a Triangle Teaser with a side total which is any number we choose?
  • How about a side total which is a fraction?
Belinda Rayment, St. Francis of Assisi, Calwell, chose to extend her students working on this task with this Investigation Guide.

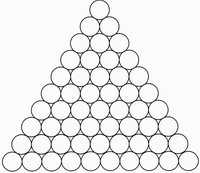
  Note: A large version of the picture on the left can be used to build a recording sheet for the students. Click on the picture to show a large version on screen, then right click (click and hold in Mac) to reveal a menu which will allow you to save the picture to your computer. Create your record sheet by inserting the picture into a word processing document.
(A large PDF version is available in the Maths At Home link below).

Note: This investigation has been included in Maths At Home. In this form it has fresh context and purpose and, in some cases, additional resources. Maths At Home activity plans encourage independent investigation through guided 'homework', or, for the teacher, can be an outline of a class investigation.

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

The paper plates make a great public display on your floorboard. The class can gather around and all be involved by having a plate each to include one by one as the rows are built.

  • How many plates will we need for the next row?
  • Can you predict the total number of plates when we make that row?
Back at their tables, students use recording paper and counters to make a table top version of this class model and explore the general question above.

Hand out another (perhaps different colour) plate to each student. Ask them to use a marker pen to trace the circumference of the base of the plate (just inside the frilly bit). Then assign each student a number and ask them to use the marker to write that number as big as possible in the circle. Provide scissors so they can carefully cut away the frilly bit. 28 students implies 28 numbers and a 7 row triangle. Fast finishers can easily supply the extra 8 numbers (20 - 36) to allow you to explore the number patterns and puzzles suggested in the task. There is a good chance your art teacher will be happy to receive the frilly cut aways.

At this stage, Plate Triangles does not have a matching lesson on Maths300.

Visit Plate Triangles on Poly Plug & Tasks.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

The Plate Triangles task is an integral part of:

  • MWA Pattern & Algebra Years 5 & 6

Green Line
Follow this link to Task Centre Home page.