Fraction Magic Square

Task 37 ... Years 4 - 10

Summary

Students usually only experience Magic Squares using whole numbers. This task shows that can also be made using fractions and opens the door to (a) linking with the classic whole number case, and (b) realising that a magic square can be made to total any number at all.

This cameo has a From The Classroom section which shows sample journal work from students in a Year 5 in England.

 

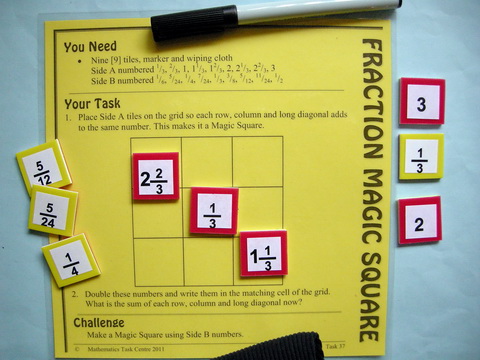
Materials

  • Two sets of 9 tiles, each with specific fraction values, and a blank 3x3 grid
  • Marker and cloth

Content

  • basic number skills
  • equivalent fractions
  • addition of fractions
  • problem solving strategies
Fraction Magic Square

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. 		   

Students who approach this task with the strategy of guess and check, will need further guidance. One question which might produce more effective investigation is:

Have you seen a similar problem?
If students mention that they have seen whole number versions then that connection can be refined. If the students have not met the classic version, then that is more likely to be the relevant starting point.

Once the classic has been experienced two approaches are possible.

  1. Can the numbers of this new task be related to the numbers of the classic?
  2. Can the strategy for solving the classic be applied to this new problem?
Writing out the fractions in their improper form creates the link with the classic magic square, ie:
1/3, 2/3,3/3, 4/3, 5/3, 6/3, 7/3, 8/3, 9/3
  • Hmm, could it be that the numbers in the classic have simply been divided by 3?
If so, the solution could be found from the solution of the classic by keeping the classic arrangement and dividing each term by 3.
  • What does this imply about the magic total of the fraction magic square?

The strategy to solve the classic problem has two stages:

  1. First, looking for unstated information to find the magic total. The total of all the numbers (in the classic) is 45. This amount has to be equally shared over 3 rows (or columns), so each row must total 15.
  2. Second, break the problem into smaller parts. Make the rows to equal the magic total. Then, shift tiles around within their rows until the columns also total the magic number. Finally, change the order of whole rows and/or whole columns until the diagonals also add to the magic total.
Find out more about the classic problem in the Magic Squares cameo.

The second set of fraction tiles is provided so students can apply what they have learnt to a new (apparently more difficult) case. However, with a little encouragement they can try to create another, and then another, fraction magic square of their own. Soon it becomes reasonable to ask:

  • If I give you any magic total can you create a magic square?
  • Is there more than one way to do the ones you create?

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

The easiest way to turn this task into a whole class investigation is to ask students to tear a piece of paper into 9 pieces and write on each of them one number from the list you put on the board. The investigation involves making the first fraction magic square, relating this to the classic example and setting up a 'Magic Square Shop' where students undertake to make the pieces of a magic square to form any total given by the customer. There are extensive notes on Maths300.

For more ideas and discussion about this investigation, open a new browser tab (or page) and visit Maths300 Lesson 72, Fraction Magic Square.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

The Fraction Magic Square task is an integral part of:

  • MWA Number & Computation Years 7 & 8

The Fraction Magic Square lesson is an integral part of:

  • MWA Number & Computation Years 7 & 8

From The Classroom

St. Edmund's Junior School, England

Matthew Reames
Year 5 		Matthew has submitted these sample journal entries about Fraction Magic Square. He comments that Ethan's explanation of why the whole numbers could not share the same rows or columns was "his question, his investigation, his solution!".
Lucy
 Ethan

Green Line
Follow this link to Task Centre Home page.