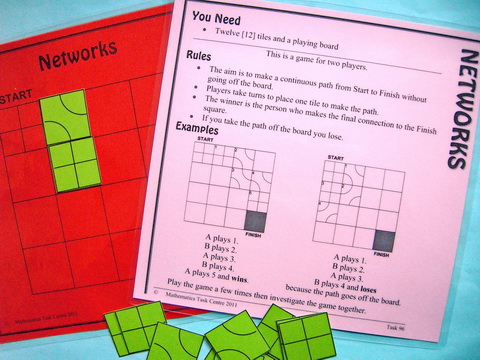
Networks

Task 96 ... Years 4 - 8

Summary

A game using simple equipment that has both a logic and spatial component. It is easy to start and easy to play, but, as always, there is an iceberg of possibilities to explore.

This cameo has a From The Classroom section which has many photos of primary student work including an outstanding 'quilt' made with network tiles by class cooperation. It also includes in the Iceberg section a link to a video of a five year old exploring this game.

 

Materials

Content

  • game analysis
  • aspects of transformation geometry
  • tessellation
  • mathematics in design
Networks

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card.

   

Note: The 12 tiles are laid out where both players can share them. Either player can choose either type of tile on any move.

Teachers often find that students really get into this game once they 'get the hang of it'. Discussion begins about strategies and thinking ahead moves. To encourage this ask students think about the:

  • minimum number of steps from Start to Finish - 3 down and 3 right - which uses 5 tiles.
  • effect of a move up or to the left - at some point it will have to be countered with a move down or right.
  • difference between the two types of tile...
    • Straight tile only allows you to stay in the column/row of the placement.
    • Curved tile allows you to stay in the column/row of placement or move through to the next column/row.
    To encourage further analysis suggest the hypothesis that if the world's best players are competing in this game then the player who plays first will win. Invite students to report on their investigation of this hypothesis.
Before exploring extensions, students might benefit from watching Piper Explores Networks, a video made with a five year old. It is remarkable how much she is working like a mathematician. Just pausing their own work to observe someone else exploring the game is likely to generate new thoughts in your students.

Extensions

A. Within the game context

  • What happens if the cards are shuffled and dealt out face down? Players have to play with the top tile of their pack on each move.
  • What happens if Player A uses only one type of tile and Player B uses the other?
  • What happens if you design your own tiles that include only the corners and midpoints of the square tile? For example:

  • What happens if the board is another size?
  • What happens if you use an equilateral triangle (or hexagon) playing board and triangle (or hexagon) tiles?
B. Beyond the game context
  • Use the tiles and make a pattern for a tiled floor. Record your pattern and extend it.
  • How many different patterns can you make?
  • What happens if you colour sections of your tiles, eg:

  • What happens if you use tiles that are divided into thirds along their edges? Blanks are supplied above. See sample photos on the left which are of creations by teachers in training at Högskolan, Malmö, Sweden
There is a project possibility here based around designing your own tiles and laying them in alternative floor patterns. See From The Classroom below.
Note: This investigation has been included in Maths At Home. In this form it has fresh context and purpose and, in some cases, additional resources. Maths At Home activity plans encourage independent investigation through guided 'homework', or, for the teacher, can be an outline of a class investigation.

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

To turn this task into a whole class investigation you will need lots of tiles. Masters for these are provided above. Print onto thin coloured card and laminate before slicing if you want to reuse the tiles in future classes.

You, or the students, can easily make your own playing boards using the Table option in Word or other word processors. The cells need to be 3cm square. Cells for the squares with edges marked in thirds are 4.5cm square.

If you want to use software more extensively, drawing programs will allow students to create and colour tiles and lay them on the screen as translations, rotations and reflections. Perhaps your Interactive Whiteboard software will also be useful.

Alternatively one copy of the task is sufficient for a whole class investigation if it is included with other tasks in a unit of work as described for Task 95, Reflections.

At this stage, Networks does not have a matching lesson on Maths300.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

Networks is not in any MWA kit. However it can be used to enrich the Space & Logic kit at Years 5/6 and Years 7/8.

From The Classroom

Camberwell Primary School

Year 6 		The school chose Patterns Around Us as their theme for National Numeracy Week. One of the activities Level 4 students were involved in was creating their own Pattern Tiles...

         

         

which they then combined into tiling patterns...

         

         

         

This was the opening session of the week. Children (and teachers) were so excited by the possibilities of these earlier trials that they decided to start again with their own designs and add colour. They measured (6cm x 6cm) and cut up their own tiles. Then made their pattern tiles and stuck each piece together to make an individual pattern. Two classes then stuck all the tile patterns together and by the end of the week they had created this stunning 'quilt'. Now the Patterns Around Us were their own.

Check Task 95, Reflections, for more work on transformations created during this week, this time using Poly Plug as the tool.

Green Line
Follow this link to Task Centre Home page.