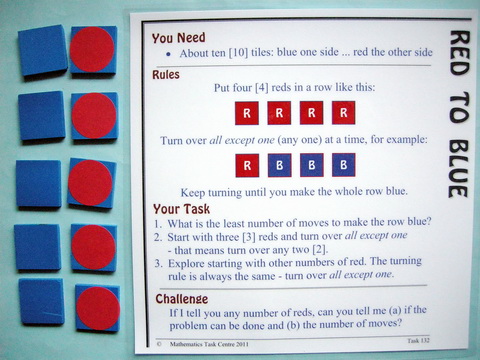
Red To Blue

Task 132 ... Years 4 - 10

Summary

It seems easy enough. The tiles have a different colour on each side. All you have to do is start with them all red and turn them so they are all blue. The only rule is that all except one must be turned over on each move. When, or if, you can do it, what is the least number of moves? Can you explain why this is the least number? What happens if we change the number of tiles? What happens if we change the turning rule?

This cameo has a From The Classroom section which shows journal entries from a Year 8 class tackling a whole class investigation for the first time. In the Iceberg section below you will also find a link to a Cube Tube video of a Year 6 group explaining their solution.

 

Materials

  • About 10 double-sided tiles

Content

  • odd and even numbers
  • problem solving strategies
  • recording and communicating mathematics
  • tree diagrams and flow charts
  • algebraic notation
  • generalisation
Red To Blue

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. 		   
Note: Colours of counters supplied with the task may vary, so, for example, your version may involve changing red to yellow.
Try the problem for yourself. It's not so easy. Students often think they have 'done it', but when you ask them to show you, they have actually lost track of their starting arrangement and have moved from all red ... to all red. So, the first level of learning with the problem is to be able to confidently demonstrate the solution. The minimum set of moves is:
R R R R
R B B B
B B R R
R R R B
B B B B
Four moves, but it looks far easier written like this than it actually is.
  • Bella, Emma, Amy & Beatrice, Year 6, Camberwell Primary School, have provided a video to Cube Tube which describes their insightful solution.

The next level of learning to explain to someone else how to do it. This could be:
  • orally, but with hands behind the back
  • in words as a set of instructions
  • as a diagram of some sort, perhaps a tree diagram
  • or...
But why encourage students to do this? Well from an educational point of view the demands of justifying to someone else have been shown to enhance the justifier's learning and enhance their literacy . And from the point of view of learning to work like a mathematician, it is simply a demand of a mathematician's work that they are able to explain their work to colleagues. 		Tree diagram focussed on the colour
not turned each time,
which must be either red or blue.

The iceberg of the problem unfolds further when we ask: What happens if we change the number of tiles? but keep the rule of all except one being turned.

It might be sensible to try smaller numbers of tiles first.

  • 1 tile ... can't do it, because the rule prevents any movement
  • 2 tiles ... too easy; turn over 1, then turn over the other, so 2 moves
  • 3 tiles ... try it for yourself; but you won't be able to do it
  • 4 tiles ... 4 moves
Predict and check for 5, 6, 7... tiles. There is a pattern. Can you describe it?

But then, what happens if we change the turning rule? For example, turn over all except two instead of all except 1?

Note: This investigation has been included in Maths At Home. In this form it has fresh context and purpose and, in some cases, additional resources. Maths At Home activity plans encourage independent investigation through guided 'homework', or, for the teacher, can be an outline of a class investigation.

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

It's so easy to state and start Red To Blue. Ask four students to stand in front of the class facing everyone. The other students are asked to name all except one of the students on each turn to do a 180. The objective is that all four students will be facing away from the class.

Once demonstrated ask the students to repeat the problem themselves using materials on the table top. If you have Poly Plug, these are perfect, but if not, ask students to tear four small squares of paper and put a cross on one side of each. Then follow the lesson through guided by the iceberg above.

For more ideas and discussion about this investigation, open a new browser tab (or page) and visit Maths300 Lesson 127, Red To Blue, which includes Classroom Contributions from a small, multi-age, one-teacher rural school and a large secondary college, as well as donated software from George Dimitriadis. George has also supplied software for Task 142, Tower of Hanoi.


Maidavale State School, Queensland: Investigating Yellow to Blue after trying it with people.
The whole school - 12 students Years 1 to 7.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

The Red To Blue task is an integral part of:

  • MWA Pattern & Algebra Years 9 & 10

The Red To Blue lesson is an integral part of:

  • MWA Space & Logic Years 5 & 6
  • MWA Space & Logic Years 7 & 8

From The Classroom

Lilydale High School

Marcus Whitby & Team
Year 8 		The school decided to build its curriculum around learning to work like a mathematician and began a combined professional development and resource program to establish the new core curriculum. The class in this lesson was experiencing their first whole class investigation as part of the PD program. The lesson was firmly set in the Working Mathematically context and followed the outline above, including using Poly Plug.

One teacher led the lesson and others observed the students. In the later debriefing discussion Marcus, Head of Department, commented on his surprise at the willingness of students to make entries in their journal. Representative entries are shown here. You will notice not only that the writers clearly understood the initial problem and were able to solve it, but that each explanation has a unique twist and some explanations make it clear, as a journal should, that there are further questions to investigate.

It should also be noted that within one 48 minute lesson, the problem was introduced, explored, solved, solution demonstrated with hands behind back as above, journalised and left open for further investigation.

Green Line
Follow this link to Task Centre Home page.