
Rectangle of SquaresTask 138 ... Years 4 - 10SummaryThe wooden squares can be arranged to form a rectangle. Easy to state, easy to start, but not so easy to do. Reasoning the problem through, rather than using guess and check, involves applying the concept of area. The task also helps to develop spatial perception. |
Materials
Content
|

IcebergA task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. |
You might like to place a removable sticker over the length in the hint, so the students experience a greater challenge. Many will accept the challenge because they know they can peek if the challenge seems too great. With or without a sticker, the first thing to do to lift the search for a solution above guess and check, is to calculate the area of the rectangle. It won't be immediately obvious to all that this will be the sum of the areas of the squares. In fact, since area and perimeter are misunderstood by some students, it may also not be obvious to some that the area of each square can be calculated from its side length. This phase may be an opportunity for point of need teaching about these concepts. The sum of the areas of the squares = 1056 sq cm. So the numbers which measure the length and width of the mystery rectangle must multiply to equal 1056.
And there is, at least, one other restriction. Both sides of the rectangle must be at least 18cm, because this is the side length of the largest rectangle. The search for two numbers whose product meets these conditions might lead to an exploration of the prime factors of 1056: 2 x 528 ... noSo, here is a place to start. We might be trying to use the squares to make a 32 x 33 rectangle. Note: 32 x 33 is not the only factorisation of 1056 that could work. For example if we begin by extracting 11, the highest prime factor, first, we get: 11 x 96 ... no32 x 33 does eventually yield a solution, although knowing these dimensions doesn't necessarily mean it comes easily.

Is there another solution? We don't know. One way to find out would be to first decide all the pairs of factors that could work and then try to make the appropriate rectangle. Is there another set of squares that makes a rectangle. Yes. Since all squares are rectangles the simplest case is:
A little trivial perhaps, but it starts off the next simplest case which is:
But that implies an infinite number of solutions because every rectangle can be composed of unit squares. Can we continue to build rectangles from squares but use different size squares? Yes ... place a 2-square above or below the two unit squares.

Now a 3-square left or right of the current model. But let's be consistent and continue to place in the anti-clockwise direction that has been established by the placements above.

Now a 5-square...

...and an 8-square...

...and so on forever!! Every step is a rectangle created from squares - all different except for the unit squares - and they create a visual pattern that spirals outwards. Wherever there is a visual pattern, there will be a number pattern. What are the numbers of these squares?
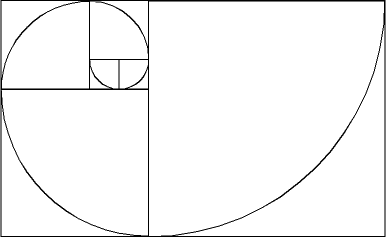
|
Whole Class InvestigationTasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. |
There is so much mathematics to explore in this task and so many opportunities to model working like a mathematician as illustrated above, that it is worth making a class set of the squares in the task. They don't have to be made from wood. Use stiff card. With a little care in handling and storing, the set will last for years. Once the original problem is solved, the work of checking other 'could be' factors could be shared among teams. Graph paper can be used to explore the Fibonacci extension. Perhaps it will turn out that even though Fibonacci is a famous mathematician, the unknown person who discovered the original rectangle of squares problem was very special too. After all, it may be the only rectangle of squares in which every square is a different size. At this stage, Rectangle of Squares does not have a matching lesson on Maths300. |
Is it in Maths With Attitude?Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. |
The Rectangle of Squares task is an integral part of:
|