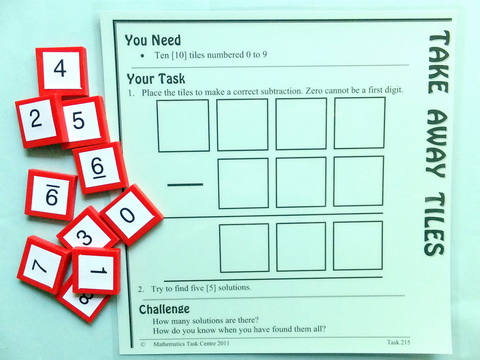
Take Away Tiles

Task 215 ... Years 4 - 10

Summary

Use all the digits 0 - 9 once only and place them in the frame to make a correct subtraction of a three digit number from a four digit number.
  • How many solutions are there?
  • How do you know you have found them all?
One of a family of digit puzzles that includes Truth Tiles 1, Crosses, Steps, Fay's Nines and Number Tiles. This one is possibly the most challenging, perhaps because students tend to be less confident with subtraction than addition. Perhaps that is a clue. Working backwards might be a worthwhile approach to the problem. 		 

Materials

  • 10 tiles numbers 0 to 9 and a frame

Content

  • algebra, generalisation in words & symbols
  • arithmetic, addition / subtraction
  • concept of proof
  • mental arithmetic
  • numbers, properties & laws
  • place value
  • reasoning
Take Away Tiles

Iceberg

A task is the tip of a learning iceberg. There is always more to a task than is recorded on the card. 		   

Students are likely to begin this task using the strategy of guess, check and improve. The important part of this strategy is the 'improve'. Each guess is a potential contribution to realising that there are limits within the problem. For example students might realise that the thousands digit must be 1.
Reason

The largest number that can be taken away is 987, so if the thousand digits is larger than 1, the subtraction would result in a four digit number not a three digit number.
As they continue to guess, check and improve, they might also realise that there are limits on where the 0 can be placed.
  • It can't be placed in the ones or tens places of the number being subtracted. If it was then 'something minus zero would equal something and we don't have two somethings the same'.
  • It can't be placed in the hundreds place of the number being subtracted either, because then it would be a two digit number instead of a three digit number.
  • And for the same reason, it can't be in the hundreds place of the answer line.
  • Also, to get a 0 in the tens or ones place of the answer the two digits above have to be the same, and we don't have two digits the same to use, so there can't be a 0 anywhere in the answer line.
So now we know where the zero can't go, the search for where it can go is reduced. In fact, it might only go in the hundreds, tens or ones places of the four digit number.

Place value is also beginning to have prominence in the investigation which leads to another key realisation, namely that there can be 'carrying' between columns. Depending on whether or not students have investigated Task 43, Number Tiles, they sometimes ask, usually when they reach a point of frustration:

Are we allowed to carry?
What does the card say about that?
Nothing.
What is the card expecting you to do?
A take away.
Do take aways sometimes have carrying?
Yeah ... oh, so we can.
Making use of all these insights soon leads to solutions that have been found by trial, record and improve. These are a considerable distance from being randomly guessed. It is important to encourage students to articulate what they were thinking when they found a solution and, having articulated, to encourage some form of written record in their journal.

Challenge

Finding just one solution will be considered a success for some students. Finding five, provides enough data to accept the challenge of hunting for all the solutions. For example, if these are the five solutions (there are other possibilities):

1062
- 479
  583 		1206
- 347
  859 		1053
- 764
  289 		1305
- 879
  426 		1089
- 456
  732

One approach to finding all the solutions might be, as suggested by the analysis above, to break the problem into smaller parts and then try every possible case. For example, there are two examples in this data that have zero in the hundreds place of the four digit number. But is that all the possible solutions with zero in this place? The students could now keep the one and zero fixed and try every other possibility for the tens and ones places. For example, begin with:

1092, 1093, 1094, ... 1098
1082, 1083, 1084, ... 1087, 1089
...
That's only 8 x 7 = 56 tests and many of these will be quickly dismissed. When all these are tested, test all possibilities with 0 in the tens place of the four digit number and then hunt for solutions when it is in the ones place. In total there are 10 unique solutions, each of which has a 'family' that can be derived from it by switching digits between the subtracted number and the answer. All these families have 8 members except the two 1089 solutions which have an extra 8 each. In total therefore, 96 solutions.

Whole Class Investigation

Tasks are an invitation for two students to work like a mathematician. Tasks can also be modified to become whole class investigations which model how a mathematician works. 		   

A great way to begin this investigation is for the students to each tear a piece of paper into five rough squares or rectangles. Then, working in pairs, they number them 0 to 9. You, as the teacher, need a set of cards about 20cm x 20cm numbered the same way. You might also want something to represent a subtraction sign and perhaps something, like a metre ruler, to 'rule off' the subtraction question from its answer.

Bring the students to a floor or central table place where they can all see and randomly hand out your cards. (They leave their paper digit cards on their tables).

  • Choose four of the students and ask them to put their cards down to make a 4 digit number.
  • Ask all the students to read the number and insist this is done as a place value statement not a string of digits, eg: 'One thousand and eighty-nine' not 'one zero eight nine' or worse 'one oh eight nine'.
  • Ask two or three more times for the digits to be moved to create another four digit number and again ask for each one to be read.
  • Choose three more of the students with cards and ask them to place their cards to make a 3 digit number under the 4 digit one.
  • Again read, switch digits within the number and read again a couple of times.
  • Place your subtraction sign and your ruler and say something like:
    Now, if we have put the cards down correctly, the cards that haven't been placed yet can be made into the answer to this take away.
Spend a little time allowing the students to jiggle the cards around to find a solution. (If one is found, which happens occasionally, record it and include the initials of the students who achieved it.) Then, when they are obviously hooked, make a statement like:
Well, I assure you it can be done. You have your own number cards on your table - you just made them. Let's see which pair is the first to find a solution.
When a solution is found ask the students to record it on the board and add their initials. Then suggest that there is yet another solution so the search can begin again. Very soon there will be reason to discuss the points in the iceberg above and before long enough solutions on the board to begin the discussion about finding all solutions. Once it has been agreed that the zero can only be in one of three places, the class can be divided into three groups, each with a leader, and with each group charged with testing all the possibilities with zero in a given place.

These days, professional mathematicians work in groups or teams most of the time.

For more ideas and discussion about this investigation, open a new browser tab (or page) and visit Maths300 Lesson 102, Take Away Tiles which includes companion software for exploring every possible case. It also begins the search for all solutions by noticing that the four digit numbers which work all have digits which sum to 9 or 18. For more senior students, this is extended to prove algebraically that this must be true and that other digit sums cannot lead to a solution.

Is it in Maths With Attitude?

Maths With Attitude is a set of hands-on learning kits available from Years 3-10 which structure the use of tasks and whole class investigations into a week by week planner. 		   

The Take Away Tiles task is an integral part of:

  • MWA Number & Computation Years 9 &10

The Take Away Tiles lesson is an integral part of:

  • MWA Number & Computation Years 9 &10

Green Line
Follow this link to Task Centre Home page.