 |
To get the best from a Task Resource, it is essential that the tasks are integrated into the curriculum. Once in a while ad hoc use has little educational value.
The photo reflects the original concept of integrating tasks by establishing a Task Centre Room devoted to practical hands-on problem solving in mathematics. Over more than three decades teachers have added many other ways to integrate tasks. Their efforts are recorded below.
|

A task centre room - the original model.
|

| Typically integration begins in a small way, perhaps with a two or three week unit. Planning for success in this way develops enthusiasm in teachers and students which generates further planning, fresh approaches and, eventually, revision of the curriculum.
Curriculum review using the Working Mathematically Process, the process of learning to work like a mathematician, as the central purpose, or core curriculum, has been very useful to many schools.
A young person learning to work like a mathematician might be compared with a young person learning to drive:
- The Learner Driver needs experience with a teacher who models how to drive well and an invitation to practice the model for themselves.
- Similarly, a Learner Mathematician needs experience with a teacher who models how a mathematician works and an invitation to apply the model for themselves.
- Tasks offer the invitation to students to work 'independently' as mathematicians and they have been included in our set because they can each be converted to a whole class investigation that models Working Mathematically.
- This page records some of the ways teachers have integrated these two uses.
Equally important in successful curriculum planning is conscious, deliberate choice of teaching craft which encourages learning. Many teachers have found this Features Checklist helpful when planning.
During the decades of Task Centre history, teachers have developed many models and structures for building curriculum around the Three Lives of a Task. Several include alternative assessment practices. Choose from those opposite, or develop and contribute your own.
|
 |
Models & Structures
- Maths With Attitude
Teaching manuals listing, exploring and structuring the use of Tasks and Maths300 lessons for 25 weeks of integrated, investigative work at every year level from 3 to 10. There are 16 manuals across these levels and each one is built around a core curriculum of learning to work like a mathematician in happy, healthy, cheerful, productive, inspiring classrooms. Many of the models and structures below are built into these manuals.
- Working Mathematically with Infants
A resource kit for Years K - 2 (five to seven year olds) with 10 weeks of planning in number for each semester of each year level - 60 weeks planning in all. Planning allows for whole class, small groups, individuals and work stations.
- Mathematician Teams
Especially useful when you and/or the class are getting used to working independently with tasks. Almost all mathematics since WWII has been discovered by teams of mathematicians, so use groups of 4 and reduce the number of tasks 'on the tables' at one time. Each team member takes a role: Reader, Counter, Questioner, Recorder. The Recorder keeps a Team Journal and each member has a diary/scrapbook of their own.
- Pass On Problem Solving
Work in teams of two, or two pairs. Each team then has a Section A and Section B. The model highlights peer teaching and gives time for every member to be both teacher and learner, as well as time to work together to take a problem further.
- Buddy System
Generally speaking the textual demands of task cards are too great to allow infant students to work independently on tasks. Using a Buddy System with senior primary students as tutors overcomes this problem and builds a richer whole school environment.
- Mixed Media Model
Begins the week with a whole class investigation then moves to three work stations - Tasks, Software, text or worksheet - each with content related to the content of the lesson. A multiple intelligences approach to exploring a topic.
- Replacement (3-Part) Unit
Called a Replacement Unit because it replaces three weeks of the current curriculum with an integrated use of Tasks and whole class investigations in the strand of the curriculum. In the first week students work from a menu of Tasks. In the second the teacher leads the exploration of one task in depth for as many as four lessons. In the third week, students select one task from the menu and, with the support of an Investigation Guide, dig deeper and prepare a report.
- Menu Maths
Learning to work like a mathematician? First give me an interesting problem. Choice! that's the centre piece of this model which, just as in a restaurant, offers a menu constructed from the best quality mathematical items available in the topic.
- Self-directed Maths Journey
An alternative 'choice' structure that sets up a mathematical landscape within the classroom and invites the students to enter it imagining that they are Explorers. Drawing on the practice of all explorers of keeping a diary the model has a vigorous literacy thrust. It works especially well in primary classrooms.
- Home/School Lending
Build a bridge between home and school using Tasks as the centre of maths around the kitchen table. Develop a three way 'conversation' between student, teacher and parents through the use of mathematics journals.
- Poster Problem Clinic
Designed decades ago as a starting point for a lesson in a Task Centre, this model focuses the classes attention at the beginning of the lesson on the mathematician's Strategy Toolbox, which will later be needed when investigating Tasks. The model is now widely used in any general classroom because every maths lesson should be calling on the Strategy Toolbox.
- Puzzle Olympics
This is a special event model. Set aside a half day and use the model when the Olympics are on, or annually with the school athletics carnival or National Mathematics Day, or monthly as an inter-class league. The link provides everything you need - a Poster Problem, a points system assigned to tasks, suggestions for a whole class starter task and more.
|
 A scope and sequence planner in each manual of the 16 kits details integrated use of the resources. Created by teachers, these kits draw on the wisdom of practice gathered over many years to provide a core curriculum centred on learning to work like a mathematician. Across the suite of kits most of the listed structures and models have been included. For more details go to our Maths With Attitude link.
Investigations - many based on tasks and adapted to the age level - are integrated with Threaded Activities from Calculating Changes to build children's number sense, concepts and skills in the context of working like a mathematician. The 60 weeks of planning builds lesson upon lesson, week upon week through each year and across the three year levels. The program is designed to link into Maths With Attitude in Year 3. For details go to Working Mathematically with Infants.
This model is built around teams of four, each of which will need one task, poster paper and broad felt tip markers. Each person also needs their Investigation Diary.
A scope and sequence planner in each manual of the 16 kits details integrated use of the resources. Created by teachers, these kits draw on the wisdom of practice gathered over many years to provide a core curriculum centred on learning to work like a mathematician. Across the suite of kits most of the listed structures and models have been included. For more details go to our Maths With Attitude link.
Investigations - many based on tasks and adapted to the age level - are integrated with Threaded Activities from Calculating Changes to build children's number sense, concepts and skills in the context of working like a mathematician. The 60 weeks of planning builds lesson upon lesson, week upon week through each year and across the three year levels. The program is designed to link into Maths With Attitude in Year 3. For details go to Working Mathematically with Infants.
This model is built around teams of four, each of which will need one task, poster paper and broad felt tip markers. Each person also needs their Investigation Diary.
- Remind students that they are learning to work like mathematicians and add that most mathematics these days is discovered by teams of mathematicians. Write the heading Mathematician Teams on the board and arrange the students in teams of four.
- Explain that mathematicians say their work begins with interesting problems and explain that students will soon be given one to explore. But first we have to assign a role to (give a job to...) each member of the team.
- Roles are:
- Counter - counts the materials when given the task and counts them back into the bag at the end. Collect any other materials needed as the team work proceeds.
- Reader - read the task card and make sure everyone understands what it says. Asks the teacher to explain further if necessary. Reminds team of words on the card if necessary while working.
- Questioner - asks questions to encourage the team to think deeper and makes sure other team members' questions are heard and explored. When the card is finished, encourages questions that extend the problem 'beyond the tip of the iceberg'.
- Recorder - keeps a record in text and diagram of the important parts of the team's work. Often uses poster size paper and markers.
|
|
- Write these job titles on the board and note that any one can contribute to any role, but the named person is the one responsible. Note that in particular that near the end of the lesson each person will be expected to make a record in their personal Investigation Diary.
- Give teams a chance to assign roles then ask all the Counters (Readers, Questioners, Recorders) to stand to confirm that each group is ready.
- Hand out the tasks to each group and ask Recorders to write its name/number and the members of the team on their poster paper. Note, this model reduces the number of tasks teachers feel they need to be familiar with at one time.
- Write the letters CRTRA down the board and explain that each team is now going to Count, Read, Try, Record, Ask as suggested in Principles of Daily Management.
- Teams begin work and teacher moves from group to group encouraging them to be as independent as possible. As necessary refer students to the Working Mathematically Process. What might a mathematician do now?
- Allow time near the end of the lesson to finalise team and personal recording. Save or display poster records as appropriate.
The focus of this model is on working with partners to explore, explain and report on an investigation. It works well with teams of either two or four. Teams of four means the teacher has fewer tasks with which to be familiar. Task Cameos support teachers to be familiar with the chosen tasks.
Each pair or four has an A person (or pair) and a B person (or pair). Teams need paper to begin a dossier about their task. Hand out the tasks and ask each team to record its name/number. This dossier remains with the task and is added to as the exploration of it continues. Allow a fixed time for the team to work on their task and record what they have found.
- At the end of this time the A people stand and pass on clockwise to the next table.
- In the next fixed time, those who remain at the table must teach the newcomers what has been learnt so far and then the new team continues to explore the problem and record.
- At the end of this time the B people stand and pass on anti-clockwise to the next table.
- In the next fixed time, those who remain at the table must teach the newcomers what has been learnt so far and then the new team continues to explore the problem and record.
- Repeat Steps 1 to 4 as time permits.
In a one hour session three pass on sequences is practical. This allows about 15 minutes work time in each sequence and various amounts of time to start and end the lesson and shuffle between groups. The process can be continued in a subsequent lesson.
As you might expect the kinaesthetic and visual nature of tasks is attractive to infant students, but the textual demands of the task card can be a barrier to independent use.
 
Depending on how a teacher organises the classroom, requests related to assistance with reading could swamp the teacher's time. A buddy system overcomes this very effectively and has additional benefits in the general school environment.
Senior students investigate tasks that Infant teachers have selected as suitable for their children. Upper school teachers, supported by the Task Cameos, encourage their students to dig into the iceberg of a task until they become 'expert'. The Upper school class discuss the importance of questioning and encouragement, rather than giving answers, and the reading assistance that may be needed. Seniors then buddy up with infants to introduce a task and lead their buddies through it as far as is appropriate.
It is important that buddies at both levels debrief with their teachers, especially following the early buddy sessions. In some schools the senior buddies take a notepad to record interesting and important things their buddies ask or do. These records make the debrief more personal.
 |
Of course, the buddy could also be a parent or grandparent. If the task is first taken home by the adult and explored, then home/school links in mathematics are strengthened. The process may even open the door to Home/School Lending.
There is much more going on here than just little kids learning some maths.
|
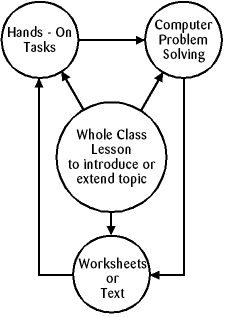 The design of a Mixed Media unit incorporates four different modes of learning into a structure which can be readily managed by one teacher, but which is enhanced when prepared and executed by a team. A three week Mixed Media Unit includes:
The design of a Mixed Media unit incorporates four different modes of learning into a structure which can be readily managed by one teacher, but which is enhanced when prepared and executed by a team. A three week Mixed Media Unit includes:
- whole class lessons
- hands-on problem solving
- problem solving software
- skill practice worksheets (or text material)
- time to reflect on learning
- assessment opportunities
If this is the first time such a structure has been used in your classroom, it is a good idea to prepare the students in a manner which 'brings them into the experiment'. Perhaps even sketch the picture at right on the whiteboard to explain how the various lessons are linked. One benefit of the structure which has been commented upon by many teachers is its allowance for the range of learning preferences (multiple intelligences) in the classroom.
A vital element of the process is to reflect on what is learned and how it is learned before the final assessment of the learning. In general the structure is run for two weeks. The third week begins with a discussion lesson around the question: What do you know now that you didn't know two weeks ago?. Responses to this discussion guide teachers in rounding off the three week unit during the remaining sessions. To extend your knowledge of your students' preferred learning styles, you might also ask the question: What activities helped you learn it?.
A Mixed Media unit can be constructed if there are tasks which relate to the topic and software that extends the topic. Use the Task Cameo Content Finder (or Task Catalogue) to identify tasks for your content purpose. The linked Task Cameos and the Maths300 search engine will help you identify whole class investigations and software which also relate. MWA Number & Computation Years 7 & 8 (see Maths With Attitude), includes details for 4 Mixed Media units constructed from these resources.
| The term 'Replacement' is used because teachers are invited to replace their existing three weeks on a topic with one of these units. In this sense, the package has a 100% professional development purpose as teachers trial and reflect on a possibly different approach to planning, classroom delivery and assessment.
A Replacement Unit is structured around twenty hands-on tasks related to the strand being studied. These are listed on a menu. It also requires Investigation Guides to extend the tasks, such as those included in the Task Cameos for Task 117 and Task 127 and others listed on the Task Cameo page.
The structure assumes that staff have arranged a Week Zero to familiarise themselves with the material and jointly plan how they will use it before beginning Week 1. |
 |
Week 1 - Introduction
- If this is the first time such an approach has been used it may be necessary to discuss the experiment with the students (...another step in our efforts to make learning mathematics a more successful experience for you...).
- In pairs, students select tasks from the menu and follow them through guided by the card. The menu is used to record the tasks tackled and journal entries can be used to record jottings as they work through the tasks.
- Teachers visit and interact with groups during the week - collecting informal assessment information and considering what the students' work suggests as the needs for the following week.
Week 2 - Formalisation
- Teacher-led lessons built on needs that have shown up in the previous week and also guided by the local curriculum objectives. These are whole class investigations which are often supported by Maths300 lesson plans.
- Given the requirements of Week 3, this second week will need to include a lesson on learning to write a maths report, if students have not had this experience.
- Assessment can be through short tests/quizzes or short-term homework assignments.
Week 3 - Investigations
- Students return to the menu and choose one task to explore for the week.
- They are led deeper into the iceberg of the task using an Investigation Guide.
- Assessment is based on an Investigation Report which becomes part of the student's Assessment Portfolio.
- Working in pairs but presenting separate reports is encouraged.
Maths With Attitude, Pattern & Algebra Year 7/8 contains a Replacement Unit plan which includes Investigation Guides for each of Years 7 & 8. Also, Maths With Attitude, Pattern & Algebra Year 9/10 includes a set of Investigation Guides.
Replacement units have been successfully used as 'in-house' professional development programs masterminded by staff members. Units have also been used as the focus of district wide professional development with a particular focus on resolving issues of transition between primary and secondary schools.
Choice. If students are to become the independent, autonomous learners we hope for them to be, we should start now. Students are capable of making their own choices about their learning. Moreover, they are enthusiastic about it.
Aaron Peeters
This quote is from an article which leads the Menu Maths section of Mathematics Centre. Aaron's article is recommended as a starting point for thinking about this model. The link continues by introducing Menu Maths Packs which support teachers to start using this model or to enrich menus they have already created. Self-directed Maths Journey and Replacement Unit, which are other structures listed on this page are also models of choice-based learning.
The concept of a Self-directed Maths Journey (SMJ) has been developed within Maths With Attitude. The description below is modified from the Year 5 & 6 Number & Computation manual. In this context the appropriate tasks are supplied in the kit, as are the appropriate lessons and software from Maths300. This manual also includes four sample SMJs. You can find this kit in our Order Form - PDF file.
Students are asked to choose their own activities within a limited, but challenging, mathematical landscape. It is just like being put into a broad, fenced environmental landscape and being given free reign to explore.
Each Self-directed Maths Journey (SMJ) is a loosely structured unit plan designed to encourage independent, self-directed mathematics learners. A feature is the development of mathematics in a language context - a model which helps teachers 'work smarter' by simultaneously achieving numeracy and literacy objectives.
Students keep a diary of their adventures and teachers encourage detailed entry in the diary by allowing sufficient time to write it. The diary has a dual purpose:
- In writing 'for an 'audience' students reprocess the work they have been doing and this helps to enhance learning.
- It provides a record that contributes to evaluation evidence.
The two week SMJ is prepared in the previous week, just as a journey into an unknown physical landscape is prepared beforehand. This occurs in two ways - by class exploration of problems which leave room for further exploration during the journey and by preparation of the diary.
Preparation of the Diary
In a mathematics session, introduce a problem (relevant to your curriculum objectives) that will need more than one session to explore its iceberg. Leave the investigation unfinished but with clear indication of the directions it can go. Explain that this investigation will be one of the activities to choose during next week's Self-directed Maths Journey.
(In the example below Bob's Buttons, Task 123, and Multo from Maths300 are used in this way.)
Follow up (in a language session?) with the concept of keeping a diary of the Self-directed Maths Journey. Collect examples of diaries from the library - literary diaries like 'Diary of Anne Frank', or professional diaries like that kept by Joseph Banks, the botanist on Captain Cook's explorations of Australia. Diaries/notebooks also feature in 'popular culture'. For example the father's diary is central to the plot in the film 'Indiana Jones and the Last Crusade', a movie well known to most students, and the film has several shots of the book showing text, sketches and maps. Note items like these as features of a diary and add others suggested by students such as the importance of dates and the possible inclusion of photographs. Encourage the use of the school's cameras to record significant mathematical moments. Consider also the possibility of an electronic diary if you are interested in achieving Information Technology outcomes in conjunction with mathematics and language.
Introduce the idea of a diary published for others, such as that of Joseph Banks, being prepared at a later time from notes taken at important moments. Perhaps use the image of a hiker travelling with a diary in their backpack and a notepad and pencil in their shirt pocket. The pad is used to record significant moments in the day and the diary is filled in later around the camp fire.
Provide 'provisions' for the SMJ:
- small spiral notepad (or equivalent)
- a more substantial book to use as the diary
- an outline of the mathematical landscape, such as the example below from MWA Number & Computation 5/6
- the Working Mathematically process page
Provide time to decorate and personalise the diary. The Working Mathematically process and the landscape page are placed in the front pages of the diary. Students are told that the next page is to be a Map Page and thereafter they record each day in text, drawing and photo as they wish.
The Map Page records each date and its activities in name only as a summary of the adventure. These items are arranged on the page as the student wishes and are linked with pathways and other landscape features in an imaginative way.
On The Journey
- Students work in pairs - it is usual to go on a journey with a companion - but keep separate diaries.
- It doesn't matter which order students tackle the activities, or which ones they tackle, or how many they tackle, or even if some activities are left started, but unfinished. What matters is that they demonstrate through their diary (and interview if you wish) how they have worked like a mathematician in each session.
- Some students may need help getting started because the range of choice may be daunting. In such cases, choose a hands-on task for them and sit down together to ask questions that will help them begin.
- Plan a compulsory teacher-led activity that students have to attend for a given number of sessions in the journey. These can be useful mini-tutorial times related to the problems, processes or skills of your chosen exploration area.
- Allow time every maths session to begin diary writing. Encourage students to work further on the diaries in their own time.
- Collect diaries regularly and annotate with encouraging comment and suggestions. Celebrate examples of student diary work that you wish to encourage in the group.
Following The SMJ
- Allow time to finalise diaries. Collect, read, comment and display.
- Ask students to prepare their own assessment of what they have learnt on the journey to add to any other assessment practice you use.
Self-directed Maths Journey II
Mathematical Landscape
- Bob's Buttons: Search for a way to predict the pattern for any given pair of groups and left overs. The software will help your search.
- Multo: Search for the best way to arrange the numbers in the grid. The software will help your search.
- What's It Worth?: Make a puzzle card.
- What's It Worth?: Try someone else's puzzle card.
- What's It Worth?: Try some puzzles from the software. Screen capture and print the results for your diary.
- Tasks: 4 & 20 Blackbirds, Add The Pack, Bob's Buttons, Change, Doctor Dart, Making Fractions 2, Number Game, Peg & Tape Fractions, Pick A Box, Steps.
- From Text: Exercises...
- Compulsory: 2 Rod Mat Chats with your teacher.
Place these items anywhere on your map page and as the unit progresses build the map of your mathematical journey.
|
Thorne Grammar, Doncaster, England has extended the Library Kit concept to make working on tasks and borrowing them to take home a regular maths period every week. Photos and details are linked here and include and include a link to the explanation of the Library Kit for Home Lending.
The Working Mathematically process requires mathematicians to dip into their Strategy Toolbox to solve problems. Task Centre teachers have developed the Poster Problem Clinic as a way making students more aware of these strategies and how they can be applied. The process begins with a large display poster, or overhead transparency, of a problem.
Learn more about this teaching technique in our Poster Problem Clinic link which also includes sample puzzles, examples of student work (Year 7) and several sources of ready-made poster problems.
The build up to the Olympic Games is a great time to bring all your tasks to a big space and create a school wide Puzzle Olympics. Teams, points for problem solving, banners, opening & closing ceremonies, medal presentations are all possible. Check the link for a considerable support, then embellish and develop it for yourself, and don't forget to send us photos and stories of your success.
Of course, you don't have to wait four years to use the idea again. Why not an Annual Mathlete Championship? Or adapting the idea to create an engaging parent night?

More from the Mathematics Task Centre
|